User:Tomruen/List of symmetric cubic graphs

In the mathematical field of graph theory, a graph $G$ is symmetric or arc-transitive if, given any two ordered pairs of adjacent vertices $(u_{1},v_{1})$ and $(u_{2},v_{2})$ of $G$ , there is an automorphism

f:V(G)\rightarrow V(G)

such that

f(u_{1})=u_{2}

and

f(v_{1})=v_{2}.

^[1]

A complete list of symmetric cubic graphs is well explored up to a certain number of vertices.

Combining the symmetry condition with the restriction that graphs be cubic (i.e. all vertices have degree 3) yields quite a strong condition, and such graphs are rare enough to be listed. They all have an even number of vertices. The Foster census and its extensions provide such lists.^[2] The Foster census was begun in the 1930s by Ronald M. Foster while he was employed by Bell Labs,^[3] and in 1988 (when Foster was 92^[1]) the then current Foster census (listing all cubic symmetric graphs up to 512 vertices) was published in book form.^[4] The list are cubic symmetric graphs with up to 1000 vertices^[5]^[6] (ten of these are also distance-transitive; the exceptions are as indicated):

Generalized Petersen graphs

7 symmetric cubic graphs are Generalized Petersen graphs, G(m,n), have 2m vertices: (4,1), (5,2), (8,3), (10,2), (10,3), (12,5), (24,5).

#C8.1	#C10.1	#C12.1	#C20.1	#C20.2	#C24.1	#C48.1
G(4,1) {4}+{4}	G(5,2) {5}+{5/2}	G(8,3) {8}+{8/3}	G(10,2) {10}+2{5}	G(10,3) {10}+{10/3}	G(12,5) {12}+{12/5}	G(24,5) {24}+{24/5}
Cubical graph	Petersen graph	Möbius–Kantor graph	Dodecahedral graph	Desargues graph	Nauru graph

Cubic distance-transitive graphs

There are only 12 cubic distance-transitive graphs.

#C4.1	#C6.1	#C8.1	#C10.1	#C14.1	#C18.1
Tetrahedral graph	Utility graph	Cubical graph	Petersen graph	Heawood graph	Pappus graph
#C20.1	#C20.2	#C28.1	#C30.1	#C90.1	#C102.1
Dodecahedral graph	Desargues graph	Coxeter graph	Tutte–Coxeter graph	Foster graph	Biggs-Smith graph

Hexagonal regular map embeddings

Cubic toroidal graphs are hexagonal regular map of the form {6,3}_b,c, with t=b²+bc+c² = (b+c)²-bc, having 2t vertices, 3t edges, and t hexagonal cycles (Girth 6). they are bipartite graphs.^[7] A hexagonal net can be drawn as a (b+c)×(b+c) array, and removing b×c array on the top corner.

When gcd(b,c)=1, they can expressed in LCF notation [n,-n]^t. (2,0) is a special case.

b\c	0	1	2	3	4	5	6	7	8	9
2	#C8.1, [5,-5]⁴, t=3 Cubical graph	#C14.1, [5,-5]⁷, t=4 Utility graph
3		#C26.1, [7,-7]¹³, t=16-3 F26A graph	#C38.1, [15,-15]¹⁹, t=25-6
4		#C42.1, [9,-9]²¹, t=25-4		#C74.1, [21,-21]³⁷, t=49-12
5		#C62.1, [11,-11]³¹, t=36-5	#C78.1, [33,-33]³⁹, t=49-10	#C98.1, [37,-37]⁴⁹, t=64-15	#C122.1 [n,-n]⁶¹, t=81-20
6		#C86.1, [13,-13]⁴³, t=49-6				#C182.1 [n,-n]⁹¹, t=121-30
7		#C114.1, [15,-15]⁵⁷, t=64-7	#C134.1 [n,-n]⁶⁷, t=81-14	#C158.1 [n,-n]⁷⁹, t=100-21	#C186.1 [n,-n]⁹³, t=121-28	#C218.1 [n,-n]¹⁰⁹, t=144-35	#C254.1 [n,-n]¹²⁷, t=169-42
8		#C146.1, [17,-17]⁷³, t=81-8		#C194.1 [n,-n]⁹⁷, t=121-24		#C258.1 [n,-n]¹²⁹, t=169-40		#C338.1 [n,-n]¹⁶⁹, t=225-56
9		#C182.1, [19,-19]⁹¹, t=100-9	#C206.1 [n,-n]¹⁰³, t=121-18		#C266.1 [n,-n]¹³³, t=169-36	#C302.1 [n,-n]¹⁵¹, t=196-45		#C386.1 [n,-n]¹⁹³, t=256-63	#C434.1 [n,-n]²¹⁷, t=17*17=289-72
10		#C222.1, [21,-21]¹¹¹, t=121-10		#C278.1 [n,-n]¹³⁹, t=169-30				#C438.1 [n,-n]²¹⁹, t=289-60		#C542.1 [n,-n]²⁷¹

Complete list 4-1000 vertices

A bipartite symmetric cubic with 2n vertices can make a self-dual (n₃) configuration, related by the Levi graph operation. The configuration will have half as many automorphisms as the cubic graph since bipartite interchange only possible after making the Levi graph.

Symmetric cubic graphs to 1000 vertices^[8]
Foster census	Vert.	Edge	Diam	Girth	s-arc trans	Aut	Aut/V	Map	Graph	Bipartite	Dist.trans
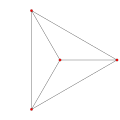
C4.1	4	6	1	3	2	24	6	{4,3}/2	Complete graph K₄, Möbius ladder M₄ Tetrahedral graph {3,3}, Hemicube graph {4,3}/2, HOG74, LCF=[2]^2	no	yes
C6.1	6	9	2	4	3	72	12	{6,3}_1,1	Utility graph, Complete bipartite graph K_3,3 Möbius ladder M₆, HOG84, LCF=[3]^3 Levi graph for symmetric configuration (3₃) and regular complex polygon 2{4}3		yes
C8.1	8	12	3	4	2	48	6	{6,3}_2,0	G(4,1), Cube graph {4,3}, HOG1022, LCF=[3,-3]^4 Levi graph for symmetric configuration (4₃) or faces of a tetrahedron		yes
C10.1	10	15	2	5	3	120	12	{5,3}/2	G(5,2) Petersen graph, HOG462, hemi-dodecahedron graph	no	yes
C14.1	14	21	3	6	4	336	24	{6,3}_2,1	Heawood graph, HOG1154, LCF=[5,-5]^7 Levi graph for Fano plane symmetric configuration (7₃)		yes
C16.1	16	24	4	6	2	96	6	{6,3}_4×2	G(8,3), Möbius–Kantor graph, HOG1229, LCF=[5,-5]^8 Levi graph for Möbius–Kantor configuration (8₃) and Möbius–Kantor polygon 3{3}3
C18.1	18	27	4	6	3	216	12	{6,3}_3,0	Pappus graph, HOG370, LCF=[5,7,-7,7,-7,-5]^3 Levi graph for Pappus configuration (9₃)₁		yes
C20.1	20	30	5	5	2	120	6	{5,3}	G(10,2), Dodecahedron graph {5,3}, HOG1043, LCF=[10,7,4,-4,-7,10,-4,7,-7,4]^2	no	yes
C20.2	20	30	5	6	3	240	12		G(10,3), Desargues graph, LCF=[5,-5,9,-9]^5 Levi graph for Desargues configuration (10₃)		yes
C24.1	24	36	4	6	2	144	6	{6,3}_2,2	G(12,5), Nauru graph, HOG1234, LCF=[5,-9,7,-7,9,-5]^4 Levi graph for Coxeter or Nauru configuration (12₃)
C26.1	26	39	5	6	1	78	3	{6,3}_3,1	F26A graph^[6], LCF=[7,-7]^13 Levi graph for a symmetric (13₃) configuration
C28.1	28	42	4	7	3	336	12		Coxeter graph	no	yes
C30.1	30	45	4	8	5	1440	48		Tutte–Coxeter graph, LCF=[-13,-9,7,-7,9,13]^5 Levi graph for Cremona-Richmond_configuration (15₃)		yes
C32.1	32	48	5	6	2	192	6	{6,3}_4,0	Dyck graph, LCF=[5,-5,13,-13]^8 Levi graph for a symmetric Dyck configuration (16₃) configuration
C38.1	38	57	5	6	1	114	3	{6,3}_3,2	LCF=[15,-15]^19 Levi graph for a symmetric (19₃) configuration
C40.1	40	60	6	8	3	480	12		LCF=[15,9,-9,-15]^10 Levi graph for a symmetric (20₃) configuration
C42.1	42	63	6	6	1	126	3	{6,3}_4,1	LCF=[9,-9]^21 Levi graph for a symmetric (21₃) configuration
C48.1	48	72	6	8	2	288	6		Double Nauru graph, G(24,5), LCF=[-7,9,19,-19,-9,7]^8 Levi graph for a symmetric (24₃) configuration
C50.1	50	75	7	6	2	300	6	{6,3}_5,0	LCF=[-21,-19,19,-19,19,-19,19,21,-21,21]^5 Levi graph for a symmetric (25₃) configuration
C54.1	54	81	6	6	2	324	6	{6,3}_3,3	LCF=[-13,-11,11,-11,11,13]^9 Levi graph for a symmetric (27₃) configuration
C56.1	56	84	7	6	1	168	3	{6,3}_4,2	LCF=[-13,-11,11,13]^14 Levi graph for a symmetric (28₃) configuration
C56.2	56	84	6	7	2	336	6	{7,3}_2,2	Cubic Klein graph, LCF=[-28,-19,-12,-18,12,15,-15,-12,18,12,19,-28,-18,18]^4	no
C56.3	56	84	7	8	3	672	12		Levi graph for a symmetric (28₃) configuration
C60.1	60	90	5	9	2	360	6		LCF=[12,-17,-12,25,17,-26,-9,9,-25,26]^6	no
C62.1	62	93	7	6	1	186	3	{6,3}_5,1	LCF=[11,-11]^31 Levi graph for a symmetric (31₃) configuration
C64.1	64	96	6	8	2	384	6		LCF=[23,-11,-29,25,-25,29,11,-23]^8 Levi graph for a symmetric (32₃) configuration
C72.1	72	108	8	6	2	432	6	{6,3}_6,0	LCF=[-31,9,-5,5,-9,31]^12 Levi graph for a symmetric (36₃) configuration
C74.1	74	111	7	6	1	222	3	{6,3}_4,3	LCF=[-21,21]^37 Levi graph for a symmetric (37₃) configuration
C78.1	78	117	8	6	1	234	3	{6,3}_5,2	LCF=[-33,33]^39 Levi graph for a symmetric (39₃) configuration
C80.1	80	120	8	10	3	960	12		LCF=[-25,9,-9,25]^20 Levi graph for a symmetric (40₃) configuration
C84.1	84	126	7	7	2	504	6			no
C86.1	86	129	9	6	1	258	3	{6,3}_6,1	LCF=[-13,13]^43 Levi graph for a symmetric (43₃) configuration
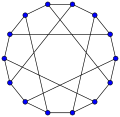
C90.1	90	135	8	10	5	4320	48		Foster graph, LCF=[17,-9,37,37,9,-17]^15 Levi graph for a symmetric Foster configuration (45₃) configuration		yes
C96.1	96	144	8	6	2	576	6	{6,3}_4,4	LCF=[-41,-39,39,41,-41,41,-41,41]^12 Levi graph for a symmetric (48₃) configuration
C96.2	96	144	7	8	3	1152	12		LCF=[-45,-33,-15,45,-39,-21,-45,39,21,45,-15,15,-45,39,-39,45,33,27,-45,15,-27,45,-39,39]^4 Levi graph for a symmetric (48₃) configuration
C98.1	98	147	9	6	1	294	3	{6,3}_5,3	LCF=[-37,37]^49 Levi graph for a symmetric (49₃) configuration
C98.2	98	147	9	6	2	588	6	{6,3}_7,0	LCF=[-43,-41,41,-41,41,-41,41,-41,41,-41,41,43,-43,43]^7 Levi graph for a symmetric (49₃) configuration
C102.1	102	153	7	9	4	2448	24		Biggs-Smith graph	no	yes
C104.1	104	156	9	6	1	312	3	{6,3}_6,2	Levi graph for a symmetric (52₃) configuration
C108.1	108	162	7	9	2	648	6			no
C110.1	110	165	7	10	3	1320	12		Levi graph for a symmetric (55₃) configuration
C112.1	112	168	7	10	1	336	3		Levi graph for a symmetric (56₃) configuration
C112.2	112	168	7	8	2	672	6		Levi graph for a symmetric (56₃) configuration
C112.3	112	168	10	8	3	1344	12		Levi graph for a symmetric (55₃) configuration
C114.1	114	171	10	6	1	342	3	{6,3}_7,1	Levi graph for a symmetric (57₃) configuration
C120.1	120	180	8	8	2	720	6		Levi graph for a symmetric (60₃) configuration
C120.2	120	180	9	10	2	720	6		Levi graph for a symmetric (60₃) configuration
C122.1	122	183	9	6	1	366	3	{6,3}_5,4	Levi graph for a symmetric (61₃) configuration
C126.1	126	189	10	6	1	378	3	{6,3}_6,3	Levi graph for a symmetric (63₃) configuration
C128.1	128	192	11	6	2	768	6	{6,3}_8,0	Levi graph for a symmetric (64₃) configuration
C128.2	128	192	8	10	2	768	6		Levi graph for a symmetric (64₃) configuration
C134.1	134	201	11	6	1	402	3	{6,3}_7,2	Levi graph for a symmetric (67₃) configuration
C144.1	144	216	7	8	1	432	3		Levi graph for a symmetric (72₃) configuration
C144.2	144	216	8	10	2	864	6		Levi graph for a symmetric (72₃) configuration
C146.1	146	219	11	6	1	438	3	{6,3}_8,1	Levi graph for a symmetric (73₃) configuration
C150.1	150	225	10	6	2	900	6	{6,3}_5,5	Levi graph for a symmetric (75₃) configuration
C152.1	152	228	11	6	1	456	3	{6,3}_6,4	Levi graph for a symmetric (76₃) configuration
C158.1	158	237	11	6	1	474	3	{6,3}_7,3	Levi graph for a symmetric (79₃) configuration
C162.1	162	243	7	12	1	486	3		Levi graph for a symmetric (81₃) configuration
C162.2	162	243	12	6	2	972	6	{6,3}_9,0	Levi graph for a symmetric (81₃) configuration
C162.3	162	243	8	12	3	1944	12		Levi graph for a symmetric (81₃) configuration
C168.1	168	252	7	12	1	504	3		Levi graph for a symmetric (84₃) configuration
C168.2	168	252	12	6	1	504	3	{6,3}_8,2	Levi graph for a symmetric (84₃) configuration
C168.3	168	252	8	8	2	1008	6			no
C168.4	168	252	7	9	2	1008	6			no
C168.5	168	252	9	7	2	1008	6			no
C168.6	168	252	8	12	2	1008	6		Levi graph for a symmetric (84₃) configuration
C182.1	182	273	13	6	1	546	3	{6,3}_6,5	Levi graph for a symmetric (91₃) configuration
C182.2	182	273	11	6	1	546	3	{6,3}_9,1	Levi graph for a symmetric (91₃) configuration
C182.3	182	273	8	7	2	1092	6			no
C182.4	182	273	9	12	3	2184	12		Levi graph for a symmetric (91₃) configuration
C186.1	186	279	12	6	1	558	3	{6,3}_7,4	Levi graph for a symmetric (93₃) configuration
C192.1	192	288	10	10	2	1152	6		Levi graph for a symmetric (96₃) configuration
C192.2	192	288	8	12	2	1152	6		Levi graph for a symmetric (96₃) configuration
C192.3	192	288	12	8	3	2304	12		Levi graph for a symmetric (96₃) configuration
C194.1	194	291	13	6	1	582	3	{6,3}_8,3	Levi graph for a symmetric (147₃) configuration
C200.1	200	300	13	6	2	1200	6	{6,3}_10,0	Levi graph for a symmetric (150₃) configuration
C204.1	204	306	9	12	4	4896	24		Levi graph for a symmetric (102₃) configuration
C206.1	206	309	13	6	1	618	3	{6,3}_9,2	Levi graph for a symmetric (103₃) configuration
C208.1	208	312	9	10	1	624	3		Levi graph for a symmetric (104₃) configuration
C216.1	216	324	9	10	2	1296	6		Levi graph for a symmetric (108₃) configuration
C216.2	216	324	8	12	2	1296	6		Levi graph for a symmetric (108₃) configuration
C216.3	216	324	12	6	2	1296	6	{6,3}_6,6	Levi graph for a symmetric (108₃) configuration
C218.1	218	327	13	6	1	654	3	{6,3}_7,5	Levi graph for a symmetric (109₃) configuration
C220.1	220	330	9	10	2	1320	6		Levi graph for a symmetric (110₃) configuration
C220.2	220	330	9	10	2	1320	6			no
C220.3	220	330	10	10	3	2640	12		Levi graph for a symmetric (110₃) configuration
C222.1	222	333	14	6	1	666	3	{6,3}_10,1	Levi graph for a symmetric (111₃) configuration
C224.1	224	336	13	6	1	672	3	{6,3}_8,4	Levi graph for a symmetric (112₃) configuration
C224.2	224	336	9	12	2	1344	6		Levi graph for a symmetric (112₃) configuration
C224.3	224	336	10	12	3	2688	12		Levi graph for a symmetric (112₃) configuration
C234.1	234	351	14	6	1	702	3	{6,3}_9,3	Levi graph for a symmetric (117₃) configuration
C234.2	234	351	8	12	5	11232	48			no
C240.1	240	360	10	9	2	1440	6		Levi graph for a symmetric (120₃) configuration
C240.2	240	360	11	10	2	1440	6			no
C240.3	240	360	10	8	2	1440	6		Levi graph for a symmetric (120₃) configuration
C242.1	242	363	15	6	2	1452	6	{6,3}_11,0	Levi graph for a symmetric (121₃) configuration
C248.1	248	372	15	6	1	744	3	{6,3}_10,2	Levi graph for a symmetric (124₃) configuration
C250.1	250	375	10	10	2	1500	6		Levi graph for a symmetric (125₃) configuration
C254.1	254	381	13	6	1	762	3	{6,3}_7,6	Levi graph for a symmetric (127₃) configuration
C256.1	256	384	9	12	1	768	3		Levi graph for a symmetric (128₃) configuration
C256.2	256	384	11	10	2	1536	6		Levi graph for a symmetric (128₃) configuration
C256.3	256	384	10	10	2	1536	6		Levi graph for a symmetric (128₃) configuration
C256.4	256	384	10	8	2	1536	6		Levi graph for a symmetric (128₃) configuration
C258.1	258	387	14	6	1	774	3	{6,3}_8,5
C266.1	266	399	15	6	1	798	3	{6,3}_9,4
C266.2	266	399	15	6	1	798	3	{6,3}_11,1
C278.1	278	417	15	6	1	834	3	{6,3}_10,3
C288.1	288	432	16	6	2	1728	6	{6,3}_12,0
C288.2	288	432	9	12	3	3456	12
C294.1	294	441	16	6	1	882	3	{6,3}_11,2
C294.2	294	441	14	6	2	1764	6	{6,3}_7,7
C296.1	296	444	15	6	1	888	3	{6,3}_8,6
C302.1	302	453	15	6	1	906	3	{6,3}_9,5
C304.1	304	456	11	10	1	912	3
C312.1	312	468	9	12	1	936	3
C312.2	312	468	16	6	1	936	3	{6,3}_10,4
C314.1	314	471	17	6	1	942	3	{6,3}_12,1
C326.1	326	489	17	6	1	978	3	{6,3}_11,3
C336.1	336	504	12	10	1	1008	3
C336.2	336	504	12	12	1	1008	3
C336.3	336	504	9	12	2	2016	6
C336.4	336	504	13	8	2	2016	6
C336.5	336	504	10	8	2	2016	6
C336.6	336	504	12	12	2	2016	6
C338.1	338	507	15	6	1	1014	3	{6,3}_8,7
C338.2	338	507	17	6	2	2028	6	{6,3}_13,0
C342.1	342	513	16	6	1	1026	3	{6,3}_9,6
C344.1	344	516	17	6	1	1032	3	{6,3}_12,2
C350.1	350	525	17	6	1	1050	3	{6,3}_10,5
C360.1	360	540	11	8	2	2160	6
C360.2	360	540	10	12	3	4320	12
C362.1	362	543	17	6	1	1086	3	{6,3}_11,4
C364.1	364	546	12	7	2	2184	6			no
C364.2	364	546	11	7	2	2184	6			no
C364.3	364	546	10	12	2	2184	6
C364.4	364	546	9	12	2	2184	6			no
C364.5	364	546	9	12	2	2184	6
C364.6	364	546	13	7	2	2184	6			no
C364.7	364	546	12	12	3	4368	12
C366.1	366	549	18	6	1	1098	3	{6,3}_13,1
C378.1	378	567	18	6	1	1134	3	{6,3}_12,3
C378.2	378	567	10	12	1	1134	3
C384.1	384	576	16	6	2	2304	6	{6,3}_8,8
C384.2	384	576	10	12	2	2304	6
C384.3	384	576	10	12	2	2304	6
C384.4	384	576	12	12	3	4608	12
C386.1	386	579	17	6	1	1158	3	{6,3}_9,7
C392.1	392	588	17	6	1	1176	3	{6,3}_10,6
C392.2	392	588	19	6	2	2352	6	{6,3}_14,0
C398.1	398	597	19	6	1	1194	3	{6,3}_13,2
C400.1	400	600	10	8	1	1200	3
C400.2	400	600	13	10	2	2400	6
C402.1	402	603	18	6	1	1206	3	{6,3}_11,5
C408.1	408	612	10	9	2	2448	6			no
C408.2	408	612	10	9	3	4896	12			no
C416.1	416	624	19	6	1	1248	3	{6,3}_12,4
C422.1	422	633	19	6	1	1266	3	{6,3}_14,1
C432.1	432	648	12	8	1	1296	3
C432.2	432	648	10	12	1	1296	3
C432.3	432	648	12	10	2	2592	6
C432.4	432	648	12	12	2	2592	6
C432.5	432	648	14	10	2	2592	6
C434.1	434	651	17	6	1	1302	3	{6,3}_9,8
C434.2	434	651	19	6	1	1302	3	{6,3}_13,3
C438.1	438	657	18	6	1	1314	3	{6,3}_10,7
C440.1	440	660	12	10	2	2640	6
C440.2	440	660	11	10	2	2640	6
C440.3	440	660	10	12	3	5280	12
C446.1	446	669	19	6	1	1338	3	{6,3}_11,6
C448.1	448	672	13	10	1	1344	3
C448.2	448	672	11	7	1	1344	3			no
C448.3	448	672	10	14	2	2688	6
C450.1	450	675	20	6	2	2700	6	{6,3}_15,0
C456.1	456	684	10	12	1	1368	3
C456.2	456	684	20	6	1	1368	3	{6,3}_14,2
C458.1	458	687	19	6	1	1374	3	{6,3}_12,5
C468.1	468	702	13	12	5	22464	48
C474.1	474	711	20	6	1	1422	3	{6,3}_13,4
C480.1	480	720	11	12	2	2880	6
C480.2	480	720	15	9	2	2880	6			no
C480.3	480	720	10	12	2	2880	6			no
C480.4	480	720	10	10	2	2880	6
C482.1	482	723	21	6	1	1446	3	{6,3}_15,1
C486.1	486	729	18	6	2	2916	6	{6,3}_9,9
C486.2	486	729	12	12	2	2916	6
C486.3	486	729	12	12	3	5832	12
C486.4	486	729	12	12	3	5832	12
C488.1	488	732	19	6	1	1464	3	{6,3}_10,8
C494.1	494	741	21	6	1	1482	3	{6,3}_14,3
C494.2	494	741	19	6	1	1482	3
C496.1	496	744	15	10	1	1488	3
C500.1	500	750	12	10	2	3000	6			no
C504.1	504	756	10	9	1	1512	3			no
C504.2	504	756	20	6	1	1512	3	{6,3}_12,6
C504.3	504	756	12	12	1	1512	3
C504.4	504	756	10	14	2	3024	6			no
C504.5	504	756	12	9	2	3024	6			no
C506.1	506	759	11	11	3	6072	12			no
C506.2	506	759	10	14	4	12144	24
C512.1	512	768	12	14	1	1536	3
C512.2	512	768	21	6	2	3072	6	{6,3}_16,0
C512.3	512	768	11	12	2	3072	6
C512.4	512	768	12	10	2	3072	6
C512.5	512	768	11	12	2	3072	6
C512.6	512	768	12	8	2	3072	6
C512.7	512	768	10	12	2	3072	6
C518.1	518	777	21	6	1	1554	3	{6,3}_13,5
C518.2	518	777	21	6	1	1554	3	{6,3}_15,2
C536.1	536	804	21	6	1	1608	3	{6,3}_14,4
C542.1	542	813	19	6	1	1626	3	{6,3}_10,9
C546.1	546	819	22	6	1	1638	3	{6,3}_11,8
C546.2	546	819	20	6	1	1638	3	{6,3}_16,1
C554.1	554	831	21	6	1	1662	3	{6,3}_12,7
C558.1	558	837	22	6	1	1674	3	{6,3}_15,3
C566.1	566	849	21	6	1	1698	3	{6,3}_13,6
C570.1	570	855	11	9	2	3420	6			no
C570.2	570	855	11	9	3	6840	12			no
C576.1	576	864	12	8	1	1728	3
C576.2	576	864	16	10	2	3456	6
C576.3	576	864	12	12	2	3456	6
C576.4	576	864	14	12	3	6912	12
C578.1	578	867	23	6	2	3468	6	{6,3}_17,0
C582.1	582	873	22	6	1	1746	3	{6,3}_14,5
C584.1	584	876	23	6	1	1752	3	{6,3}_16,2
C592.1	592	888	15	10	1	1776	3
C600.1	600	900	12	12	2	3600	6
C600.2	600	900	20	6	2	3600	6	{6,3}_10,10
C602.1	602	903	21	6	1	1806	3	{6,3}_11,9
C602.2	602	903	23	6	1	1806	3	{6,3}_15,4
C608.1	608	912	21	6	1	1824	3	{6,3}_12,8
C614.1	614	921	23	6	1	1842	3	{6,3}_17,1
C618.1	618	927	22	6	1	1854	3	{6,3}_13,7
C620.1	620	930	10	15	4	14880	24			no
C624.1	624	936	16	10	1	1872	3
C624.2	624	936	12	14	1	1872	3
C626.1	626	939	23	6	1	1878	3	{6,3}_16,3
C632.1	632	948	23	6	1	1896	3	{6,3}_14,6
C640.1	640	960	12	10	3	7680	12
C648.1	648	972	12	12	1	1944	3
C648.2	648	972	13	12	1	1944	3
C648.3	648	972	10	12	2	3888	6
C648.4	648	972	12	12	2	3888	6
C648.5	648	972	14	12	2	3888	6
C648.6	648	972	24	6	2	3888	6	{6,3}_18,0
C650.1	650	975	23	6	1	1950	3	{6,3}_15,5
C650.2	650	975	11	12	5	31200	48
C654.1	654	981	24	6	1	1962	3	{6,3}_17,2
C660.1	660	990	11	10	2	3960	6			no
C662.1	662	993	21	6	1	1986	3	{6,3}_11,10
C666.1	666	999	22	6	1	1998	3	{6,3}_12,9
C672.1	672	1008	12	12	1	2016	3
C672.2	672	1008	24	6	1	2016	3	{6,3}_16,4
C672.3	672	1008	12	12	1	2016	3
C672.4	672	1008	12	8	2	4032	6			no
C672.5	672	1008	13	12	2	4032	6
C672.6	672	1008	12	12	2	4032	6
C672.7	672	1008	12	14	2	4032	6
C674.1	674	1011	23	6	1	2022	3	{6,3}_13,8
C680.1	680	1020	10	12	2	4080	6			no
C680.2	680	1020	11	10	3	8160	12			no
C686.1	686	1029	25	6	1	2058	3	{6,3}_14,7
C686.2	686	1029	23	6	1	2058	3	{6,3}_18,1
C686.3	686	1029	12	12	2	4116	6
C688.1	688	1032	17	10	1	2064	3
C698.1	698	1047	25	6	1	2094	3	{6,3}_17,3
C702.1	702	1053	24	6	1	2106	3	{6,3}_15,6
C702.2	702	1053	14	12	1	2106	3
C720.1	720	1080	11	10	1	2160	3
C720.2	720	1080	12	8	1	2160	3
C720.3	720	1080	10	10	2	4320	6
C720.4	720	1080	12	8	2	4320	6
C720.5	720	1080	16	8	2	4320	6
C720.6	720	1080	12	12	3	8640	12
C722.1	722	1083	25	6	1	2166	3	{6,3}_16,5
C722.2	722	1083	25	6	2	4332	6	{6,3}_19,0
C726.1	726	1089	22	6	2	4356	6	{6,3}_11,11
C728.1	728	1092	25	6	1	2184	3	{6,3}_12,10
C728.2	728	1092	23	6	1	2184	3	{6,3}_18,2
C728.3	728	1092	14	12	2	4368	6
C728.4	728	1092	13	12	2	4368	6
C728.5	728	1092	12	12	2	4368	6
C728.6	728	1092	12	12	2	4368	6
C728.7	728	1092	14	12	3	8736	12
C734.1	734	1101	23	6	1	2202	3	{6,3}_13,9
C744.1	744	1116	13	12	1	2232	3
C744.2	744	1116	24	6	1	2232	3	{6,3}_14,8
C746.1	746	1119	25	6	1	2238	3	{6,3}_17,4
C750.1	750	1125	12	12	2	4500	6
C758.1	758	1137	25	6	1	2274	3	{6,3}_15,7
C762.1	762	1143	26	6	1	2286	3	{6,3}_19,1
C768.1	768	1152	12	12	1	2304	3
C768.2	768	1152	18	10	2	4608	6
C768.3	768	1152	12	14	2	4608	6
C768.4	768	1152	13	12	2	4608	6
C768.5	768	1152	12	12	2	4608	6
C768.6	768	1152	11	12	2	4608	6
C768.7	768	1152	11	12	2	4608	6
C774.1	774	1161	26	6	1	2322	3	{6,3}_18,3
C776.1	776	1164	25	6	1	2328	3	{6,3}_16,6
C784.1	784	1176	17	10	1	2352	3
C784.2	784	1176	19	10	2	4704	6
C794.1	794	1191	23	6	1	2382	3	{6,3}_12,11
C798.1	798	1197	24	6	1	2394	3	{6,3}_13,10
C798.2	798	1197	26	6	1	2394	3	{6,3}_17,5
C800.1	800	1200	27	6	2	4800	6	{6,3}_20,0
C806.1	806	1209	25	6	1	2418	3	{6,3}_14,9
C806.2	806	1209	27	6	1	2418	3	{6,3}_19,2
C816.1	816	1224	12	8	2	4896	6			no
C816.2	816	1224	12	9	2	4896	6			no
C816.3	816	1224	12	12	2	4896	6
C816.4	816	1224	12	9	2	4896	6			no
C816.5	816	1224	12	8	2	4896	6			no
C816.6	816	1224	11	12	2	4896	6
C816.7	816	1224	10	15	2	4896	6			no
C816.8	816	1224	12	9	2	4896	6			no
C816.9	816	1224	11	14	3	9792	12
C818.1	818	1227	25	6	1	2454	3	{6,3}_15,8
C824.1	824	1236	27	6	1	2472	3	{6,3}_18,4
C832.1	832	1248	19	10	1	2496	3
C834.1	834	1251	26	6	1	2502	3	{6,3}_16,7
C840.1	840	1260	11	12	1	2520	3
C840.2	840	1260	11	10	2	5040	6
C842.1	842	1263	27	6	1	2526	3	{6,3}_20,1
C854.1	854	1281	27	6	1	2562	3	{6,3}_17,6
C854.2	854	1281	27	6	1	2562	3	{6,3}_19,3
C864.1	864	1296	24	6	2	5184	6	{6,3}_12,12
C864.2	864	1296	16	12	2	5184	6
C864.3	864	1296	14	12	2	5184	6
C864.4	864	1296	15	10	2	5184	6
C866.1	866	1299	25	6	1	2598	3	{6,3}_13,11
C872.1	872	1308	25	6	1	2616	3	{6,3}_14,10
C878.1	878	1317	27	6	1	2634	3	{6,3}_18,5
C880.1	880	1320	12	10	2	5280	6
C880.2	880	1320	12	10	2	5280	6
C880.3	880	1320	12	14	3	10560	12
C882.1	882	1323	26	6	1	2646	3	{6,3}_15,9
C882.2	882	1323	28	6	2	5292	6
C888.1	888	1332	14	12	1	2664	3
C888.2	888	1332	28	6	1	2664	3	{6,3}_20,2
C896.1	896	1344	11	12	1	2688	3
C896.2	896	1344	27	6	1	2688	3	{6,3}_16,8
C896.3	896	1344	13	12	1	2688	3
C896.4	896	1344	12	12	1	2688	3
C896.5	896	1344	14	14	2	5376	6
C906.1	906	1359	28	6	1	2718	3	{6,3}_19,4
C912.1	912	1368	20	10	1	2736	3
C912.2	912	1368	12	14	1	2736	3
C914.1	914	1371	27	6	1	2742	3	{6,3}_17,7
C926.1	926	1389	29	6	1	2778	3
C936.1	936	1404	28	6	1	2808	3	{6,3}_18,6
C936.2	936	1404	14	12	1	2808	3
C936.3	936	1404	11	12	3	11232	12			no
C938.1	938	1407	25	6	1	2814	3	{6,3}_13,12
C938.2	938	1407	29	6	1	2814	3	{6,3}_20,3
C942.1	942	1413	26	6	1	2826	3	{6,3}_14,11
C950.1	950	1425	27	6	1	2850	3	{6,3}_15,10
C960.1	960	1440	12	14	2	5760	6
C960.2	960	1440	18	12	2	5760	6
C960.3	960	1440	12	14	2	5760	6
C962.1	962	1443	27	6	1	2886	3	{6,3}_16,9
C962.2	962	1443	29	6	1	2886	3	{6,3}_19,5
C968.1	968	1452	29	6	2	5808	6	{6,3}_22,0
C974.1	974	1461	29	6	1	2922	3
C976.1	976	1464	19	10	1	2928	3
C978.1	978	1467	28	6	1	2934	3	{6,3}_17,8
C992.1	992	1488	29	6	1	2976	3	{6,3}_20,4
C998.1	998	1497	29	6	1	2994	3	{6,3}_18,7
C1000.1	1000	1500	15	10	2	6000	6
C1000.2	1000	1500	13	12	2	6000	6

References

^ ^a ^b Biggs, Norman (1993). Algebraic Graph Theory (2nd ed.). Cambridge: Cambridge University Press. pp. 118–140. ISBN 0-521-45897-8.
^ Marston Conder, Trivalent symmetric graphs on up to 768 vertices, J. Combin. Math. Combin. Comput, vol. 20, pp. 41–63
^ Foster, R. M. "Geometrical Circuits of Electrical Networks." Transactions of the American Institute of Electrical Engineers 51, 309–317, 1932.
^ "The Foster Census: R.M. Foster's Census of Connected Symmetric Trivalent Graphs", by Ronald M. Foster, I.Z. Bouwer, W.W. Chernoff, B. Monson and Z. Star (1988) ISBN 0-919611-19-2
^ Biggs, p. 148
^ ^a ^b Weisstein, Eric W., "Cubic Symmetric Graph", from Wolfram MathWorld.
^ Coxeter, H. S. M.; Moser, W. O. J. (1980), Generators and Relations for Discrete Groups, Ergebnisse der Mathematik und ihrer Grenzgebiete, vol. 14 (4th ed.), Springer Verlag, ISBN 978-0-387-09212-6, 8.4 Maps of type {3,6} or {6,3} on a torus.
^ Trivalent (cubic) symmetric graphs on up to 10000 vertices. Marston Conder, 2011.

Cubic symmetric graphs (The Foster Census). Data files for all cubic symmetric graphs up to 768 vertices, and some cubic graphs with up to 1000 vertices. Gordon Royle, updated February 2001, retrieved 2009-04-18.
EDGE-TRANSITIVE CUBIC GRAPHS: CATALOGUING AND ENUMERATION MARSTON CONDER AND PRIMOZ POTOCN, 2025
A refined classification of symmetric cubic graphs Marston Conder and Roman Nedela, 2009

[biggs-1] Biggs, Norman (1993). Algebraic Graph Theory (2nd ed.). Cambridge: Cambridge University Press. pp. 118–140. ISBN 0-521-45897-8.

[2] Marston Conder, Trivalent symmetric graphs on up to 768 vertices, J. Combin. Math. Combin. Comput, vol. 20, pp. 41–63

[3] Foster, R. M. "Geometrical Circuits of Electrical Networks." Transactions of the American Institute of Electrical Engineers 51, 309–317, 1932.

[4] "The Foster Census: R.M. Foster's Census of Connected Symmetric Trivalent Graphs", by Ronald M. Foster, I.Z. Bouwer, W.W. Chernoff, B. Monson and Z. Star (1988) ISBN 0-919611-19-2

[5] Biggs, p. 148

[F26A-6] Weisstein, Eric W., "Cubic Symmetric Graph", from Wolfram MathWorld.

[7] Coxeter, H. S. M.; Moser, W. O. J. (1980), Generators and Relations for Discrete Groups, Ergebnisse der Mathematik und ihrer Grenzgebiete, vol. 14 (4th ed.), Springer Verlag, ISBN 978-0-387-09212-6, 8.4 Maps of type {3,6} or {6,3} on a torus.

[8] Trivalent (cubic) symmetric graphs on up to 10000 vertices. Marston Conder, 2011.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

Generalized Petersen graphs

Cubic distance-transitive graphs

Hexagonal regular map embeddings

Complete list 4-1000 vertices

See also

References